Title: Optimal transportation under nonholonomic constraints

Authors: A.A. Agrachev, P. Lee

Journal title: Transactions of the Amer. Math. Soc.

Year: 2009

Volume: 361

Pages: 6019-6047

Abstract:

We study Monge’s optimal transportation problem, where the cost is given by optimal control cost. We prove the existence and uniqueness of an optimal map under certain regularity conditions on the Lagrangian, absolute continuity of the measures with respect to Lebesgue, and most importantly the absence of
sharp abnormal minimizers. In particular, this result is applicable in the case of subriemannian manifolds with a 2-generating distribution and cost given by 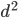$d^2$, where 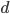$d$ is the subriemannian distance. Also, we discuss some properties of the optimal plan when abnormal minimizers are present. Finally, we consider some examples of displacement interpolation in the case of Grushin plane.

File: Скачать