We consider a two-driving wheel mobile robot with a trailer on a plane. Possible positions are given by 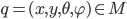 , where
, where 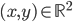 is a midpoint of the robot and
is a midpoint of the robot and 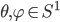 are angles of orientation of the robot and the trailer. The kinematic model reads as
are angles of orientation of the robot and the trailer. The kinematic model reads as 
where 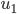 and
and 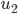 are, respectively, linear and angular velocities of the robot as controls;
are, respectively, linear and angular velocities of the robot as controls; 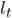 and
and 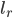 are constants defining the geometry of the hooking up system. The motion planning problem is to find controls
are constants defining the geometry of the hooking up system. The motion planning problem is to find controls 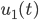 ,
, 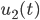 that steer (1) from a given initial configuration
that steer (1) from a given initial configuration 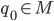 to a given final one
to a given final one 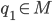 , i.e., to find a path
, i.e., to find a path 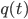 , s.t.
, s.t. 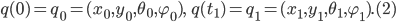
The method of nipotent approximation is used. The corresponding nipotent problem is a sub-Riemannian (SR) problem on Engel group. We propose an iterative scheme to solve the motion planning problem (1)-(2), where on each iteration the optimal controls for the nilpotent system are applied to (2). The method converges if 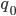 and
and 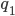 are close enough. Moreover, the obtained trajectory is close to optimal in terms of
are close enough. Moreover, the obtained trajectory is close to optimal in terms of 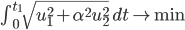 , with
, with 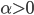 .
.
Mathematical Control Theory
with a special session in honor of Gianna Stefani
Porquerolles, June 27th-30th 2017

